 |
||||||||
|
||||||||
|
|
The world is four-dimensional - Hermann Minkowski's irrefutable proof
Note 1: The main reason for using the expression "irrefutable proof" is that experiments would be impossible if it were wrong - see below. Note 2: The strong formulation of this piece of foundational knowledge ("irrefutable proof") might look to some colleagues not sufficiently "politically correct." Although the reason for choosing this formulation seems quite transparent - to invite and even provoke colleagues to challenge the proposed piece of foundational knowledge - it is worth saying two more things:
* What appears to be a real problem in physics is that some physicists regard physical theories as nothing more than mere descriptions (which ultimately implies that they do not represent anything in the external world) and therefore issues such as the reality of spacetime are regarded as belonging to philosophy?! That is hardly physics at its best because it is only physics that can adequately address and determine the dimensionality of the world.
Galison's masterful summary of the essence of Minkowski's discovery:
"The views of space and time which I want to present to you arose from the domain of experimental physics, and therein lies their strength. Their tendency is radical. From now onwards space by itself and time by itself will recede completely to become mere shadows and only a type of union of the two will still stand independently on its own." Here is Minkowski's most general proof that the world is four-dimensional contained in his 1908 lecture. To explain the experiment of Michelson-Morley, which failed to detect the Earth's absolute motion (and all other failed experiments to discover absolute motion since Galileo), Lorentz formally suggested that observers in relative motion have different times. Einstein postulated that the times of different observers are equally good, that is, each observer has his own time, and that for Einstein meant that time is relative. As a mathematician Minkowski probably immediately realized that as observers in relative motion have different equally real times, they inescapably have different spaces2 as well, because space is defined as a set of simultaneous events and different times imply different simultaneity, i.e., different spaces (or simply - different times imply different spaces because space is perpendicular to time, if the convention ε=1/2 is used): "Hereafter we would then have in the world no more the space, but an infinite number of spaces analogously as there is an infinite number of planes in three-dimensional space. Three-dimensional geometry becomes a chapter in four-dimensional physics. You see why I said at the beginning that space and time will recede completely to become mere shadows and only a world in itself will exist." Therefore the failure of all experiments to detect absolute motion (encapsulated in the principle of relativity - physical phenomena look the same in all inertial reference frames3) has indeed a profound physical meaning - all those experiments failed to detect absolute motion (i.e., uniform motion in the absolute space) because there exists not a single (and therefore absolute) space, but many spaces (and many times) in the world; physical phenomena look the same for all observers in relative motion, because each observer performs experiments in his own space and uses his own time (e.g., the speed of light is the same for all observers since each observer measures it in his own space by using his own time). Now Minkowski's argument, deduced from the experimental evidence, that the world is four-dimensional, becomes evident: the world must be four-dimensional in order that observers in relative motion have different spaces (and times). Minkowski did not stress that the experimental results (that gave rise to the principle of relativity) would be impossible (i.e., the failure to detect absolute motion by experiments would no longer be observed and absolute motion would become detectable), if the world were three-dimensional (which would mean that there would exist a single and therefore absolute three-dimensional space and a single and therefore absolute time) most probably because it looked self-evident to him (as a mathematician).4 And, indeed, if the physical world were three-dimensional, there would exist a single (and therefore absolute) space, i.e. a single class of simultaneous events (a single time), which would mean that simultaneity and time would be absolute in contradiction with both the theory of relativity and, most importantly, with the experiments which failed to detect absolute motion. Minkowski's general argument alone is sufficient to prove that the world is four-dimensional,5 but it should be also emphasized that all experiments, which confirmed the kinematic relativistic effects, would be also impossible6; e.g., if the world were three-dimensional, there would exist a single (absolute) time, a single (absolute) space and therefore a single (absolute) class of simultaneous events which means that the experiments that confirmed the relativistic effects of time dilation and length contraction7 would be impossible because these effects are specific manifestations of relativity of simultaneity [4]. That is why, the relativistic experiments and the theory of relativity itself are impossible in a three-dimensional world. To see a specific demonstration of why the experiments which confirmed the kinematic relativistic effects would be impossible if the world were not four-dimensional (i.e., if spacetime were just a mathematical space) examine Minkowski's own explanation of length contraction (which is the accepted explanation); start at the last paragraph on p. 79.8 There are still physicists and philosophers who have been effectively refusing to face the implications of a real four-dimensional world due to the huge challenges they pose. But trying to squeeze Nature into our pre-set and deceivingly comfortable views of the world should not be an option for anyone in the 21st century.
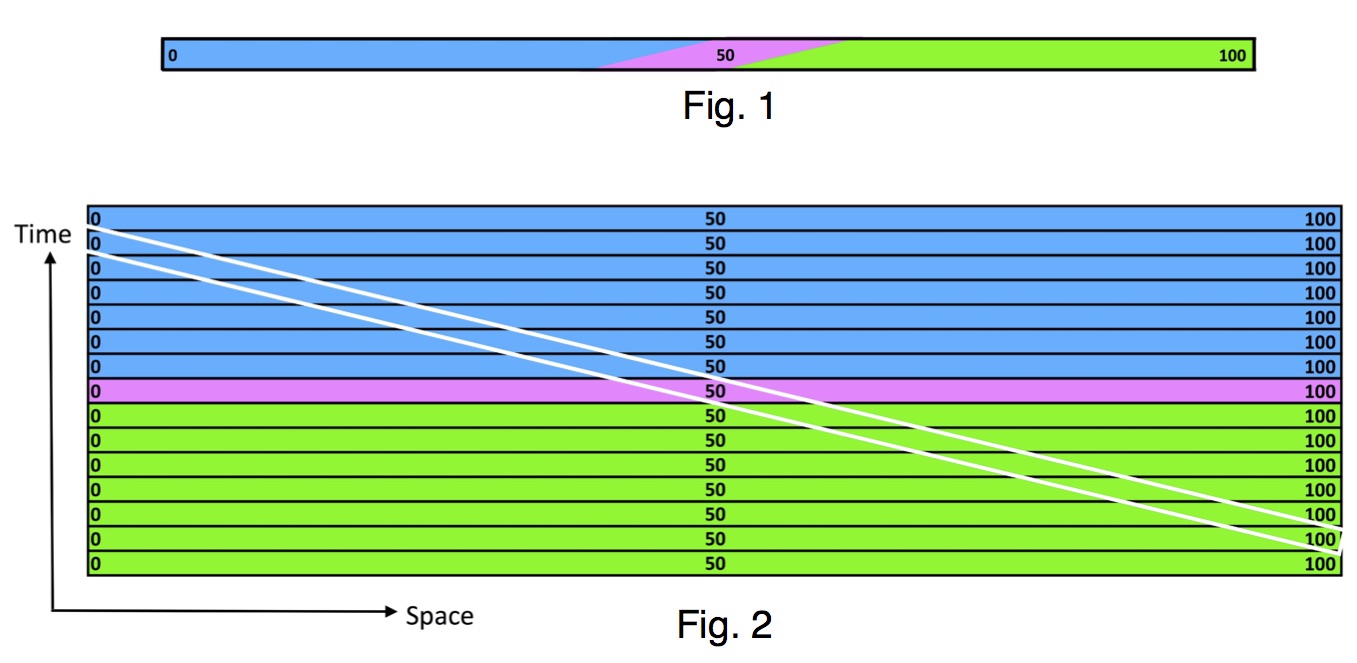
1 It was Minkowski who first realized that reality is an absolute four-dimensional world with time as the fourth dimension by successfully decoding the profound physical message hidden in the failed experiments (including the Michelson-Morley experiment) to detect absolute motion (see [4] for a detailed analysis). Poincaré first published his observation that the Lorentz transformations can be viewed as rotations in a four-dimensional space but he regarded it as a mathematical construction which does not represent anything in the physical world (see Appendix in the link in the next sentence). Also, two things appear to indicate that Minkowski arrived independently at what Einstein called special relativity and at the notion of spacetime. 2 Minkowski specifically pointed out that "Neither Einstein nor Lorentz disputed the concept of space" [2, p. 65] and provided an additional insight from his analysis of the mathematical formalism of classical mechanics (in his efforts to reveal the physical meaning of the failed experiments to detect absolute motion) that led him to the notion of an absolute four-dimensional world - die Welt (spacetime): "To step over the concept of space in such a way is an instance of what can be achieved only due to the audacity of mathematical culture. After this further step, which is indispensable for the true understanding of the group Gc, I think the word relativity postulate used for the requirement of invariance under the group Gc is very feeble. Since the meaning of the postulate is that through the phenomena only the four-dimensional world in space and time is given, but the projection in space and in time can still be made with certain freedom, I want to give this affirmation rather the name the postulate of the absolute world (or shortly the world postulate)." 3 If they did not look the same, absolute motion would be detected. 4 Those who disagree seemed to have never tried to see that that is indeed self-evident. Even worse - those, who claim that spacetime is nothing more than a mathematical space, have not asked themselves even the exceedingly obvious question - Why would a mathematician announce excitedly (see the beginning of his 1908 lecture above) the introduction of one more mathematical space. 5 Because experimental results (the experimental failure to detect absolute motion) would be impossible if the world were not four-dimensional - uniform motion with respect to the single (absolute) space of a three-dimensional world would be detected by experiment. 6 For example, it is an experimental fact, used every fraction of a second by the GPS, that observers in relative motion have different times, which is impossible in a three-dimensional world, where there are a single space and a single time. 7 Along with time dilation, the muon experiment effectively tested length contraction experimentally as well: "In the muon's reference frame, we reconcile the theoretical and experimental results by use of the length contraction effect, and the experiment serves as a verification of this effect" [3]. 8 Here are the last two figures of Minkowski's more visualized version of his explanation of length contraction (given in that text) - both the theory and the experiment (since length contraction along with time dilation are specific manifestations of relativity of simultaneity) say that the contracted meter stick (Fig. 1) must contain three colours, but this is only possible, as seen in Fig. 2, if the meter stick exists equally at all moments of its history in time, which proves the reality of the meter stick's worldtube and therefore the reality of spacetime. Minkowski did not say length contraction is impossible in a three-dimensional world (as a mathematician he did not state obvious things), but that is exceedingly clear from the two figures below (as discussed in the linked text above) and one more time explains why the adjective "irrefutable" in this page title was used.
1. P.L. Galison, Minkowski's Space-Time: From Visual Thinking to the Absolute World, Historical Studies in the Physical Sciences, 10 (1979) pp. 85-121, p. 98. 2. H. Minkowski, Spacetime: Minkowski's Papers on Spacetime Physics, translated from the German by Gregorie Dupuis-Mc Donald, Fritz Lewertoff and Vesselin Petkov; edited by V. Petkov (Minkowski Institute Press, Montreal 2020). Here is a copy of Minkowski's paper Space and Time. 3. G.F.R. Ellis, R.M. Williams, Flat and Curved Space-Times (Oxford University Press, Oxford 1988), p. 103 4. V. Petkov, "Physics as Spacetime Geometry." In: A. Ashtekar, V. Petkov (eds), Springer Handbook of Spacetime (Springer, Heidelberg 2014), pp. 141-163, sec. 8.2
|
|||||||
